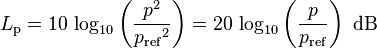sound pressure

Posted by ROSHAN B.S on Aug. 25, 2014, 6:48 p.m.

Sound pressure is the difference, in a given medium, between average local pressure and the pressure in the sound wave. A square of this difference (i.e., a square of the deviation from the equilibrium pressure) is usually averaged over time and/or space, and a square root of this average provides a root mean square (RMS) value. For example, 1 Pa RMS sound pressure (94 dBSPL) in atmospheric air implies that the actual pressure in the sound wave oscillates between (1 atm 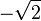$-\sqrt{2}$ Pa) and (1 atm $+\sqrt{2}$ Pa), that is between 101323.6 and 101326.4 Pa. As the human ear can detect sounds with a wide range of amplitudes, sound pressure is often measured as a level on a logarithmic decibel scale. The sound pressure level (SPL) or L_p is defined as

L_\mathrm{p}=10\, \log_{10}\left(\frac{{p}^2}{{p_\mathrm{ref}}^2}\right) =20\, \log_{10}\left(\frac{p}{p_\mathrm{ref}}\right)\mbox{ dB}\,

where p is the root-mean-square sound pressure and

p_\mathrm{ref}

is a reference sound pressure. Commonly used reference sound pressures, defined in the standard ANSI S1.1-1994, are 20µPa in air and 1 µPa in water. Without a specified reference sound pressure, a value expressed in decibels cannot represent a sound pressure level.

Since the human ear does not have a flat spectral response, sound pressures are often frequencyweighted so that the measured level matches perceived levels more closely. The International Electrotechnical Commission (IEC) has defined several weighting schemes. A-weighting attempts to match the response of the human ear to noise and A-weighted sound pressure levels are labeled dBA. C-weighting is used to measure peak levels.

Back to All Posts